Multi-Step Word Problems Using Model Method
1. A T-shirt costs $19 and a skirt costs 3 times as much.
If Annie is left with $24 after paying for the T-shirt and the skirt, how much money had she at first?
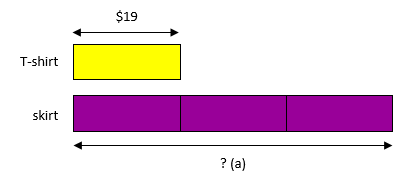 4 × $19 = $76 ......(a)
4 × $19 = $76 ......(a)The T-shirt and skirt cost $76 altogether.
 $76 + $24 = $100
$76 + $24 = $100Annie had $100 at first.
2. Ben had $15. After paying for 3 cards and some stamps, he had $3 left.
If each card cost $2 and each stamp cost $1, find the number of stamps he bought.
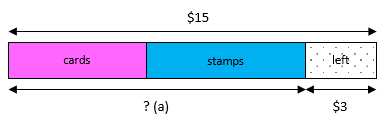
$15 − $3 = $12 ......(a)
He spent $12 on the cards and stamps altogether.
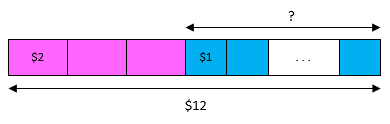
3 × $2 = $6
The cards cost $6.
$12 − $6 = $6
The stamps cost $6.
$6 ÷ $1 = 6
He bought 6 stamps.
3. Charlie bought a board game at $7 off. He also bought a water shooter and paid $41 altogether.
What was the original price of the board game, if, before discount, it cost 5 times as much as the water shooter?
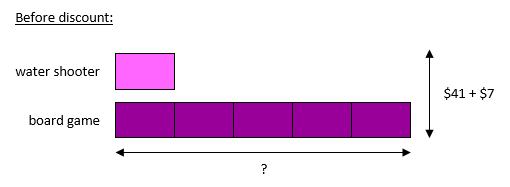
$41 + $7 = $48
The original price of the board game and water shooter was $48.
6 units −> $48
1 unit −> $48 ÷ 6 = $8
5 units −> 5 × $8 = $40
The original price of the board game was $40.
4. Dave and Eli bought some DVDs and paid $96 altogether.
If Eli bought 10 more DVDs than Dave and paid $20 more than him, find the number of DVDs that Dave bought.

$20 ÷ 10 = $2
Cost of each DVD is $2.
$96 − $20 = $76
2 units −> $76
1 unit −> $76 ÷ 2 = $38
Dave spent $38 on the DVDs.
$38 ÷ $2 = 19
Dave bought 19 DVDs.
5. Each month Li pays bills for electricity and water.
If she pays $36 a month for electricity, and $720 a year for electricity and water together, find the amount of money she pays for water each month.

$720 ÷ 12 = $60
She pays $60 a month for electricity and water.
$60 − $36 = $24
She pays $24 for water each month.
6. A supermarket is having a promotion on frozen pizzas that are normally priced at $9 each.
If Ken buys 2 frozen pizzas and gets 1 free, how much does he save on each frozen pizza in this promotion?

In the promotion, Ken pays the usual price for 2 pizzas and gets the 3rd pizza for free.
$9 × 2 = $18
Ken pays $18 for 3 pizzas.
$18 ÷ 3 = $6 ......(a)
In the promotion, each pizza is $6.
$9 − $6 = $3
He saves $3 on each pizza in the promotion.
7. Mia, Neil and Olivia had a total of $160.
Neil had $8 more than Mia. Olivia had 5 times as much money as Neil.
How much money had Mia?
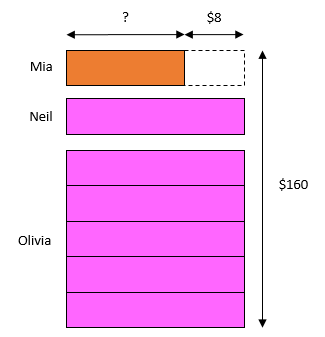
$160 + $8 = $168
7 units −> $168
1 unit −> $168 ÷ 7 = $24
Neil had $24.
$24 − $8 = $16
Mia had $16.
7 units −> $168
1 unit −> $168 ÷ 7 = $24
Neil had $24.
$24 − $8 = $16
Mia had $16.
8. Piper had 50 m of lace that she divided into pieces of 3 m each.
She sold each 3-m piece for $5 and the leftover at a discount. She collected $82 altogether.
How much did she sell the leftover lace for?

50 ÷ 3 = 16 R 2 ......(a)
She got 16 3-m pieces.
There was a leftover piece of 2 m.
16 × $5 = $80
She sold the 3-m pieces for $80 altogether.
$82 − $80 = $2
She sold the leftover piece for $2.
9. Qi had 2 times as many crayons as Maria.
After borrowing some crayons from Maria, Qi now has 5 times as many crayons as Maria.
If they have 150 crayons altogether, how many crayons did Qi borrow from Maria?
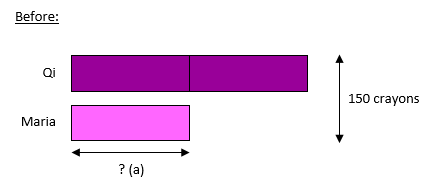 3 units −> 150 crayons
3 units −> 150 crayons1 unit −> 150 ÷ 3 = 50 crayons
Maria had 50 crayons at first. ......(a)
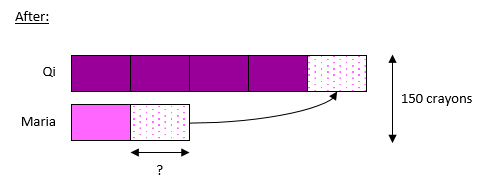 6 units −> 150 crayons
6 units −> 150 crayons1 unit −> 150 ÷ 6 = 25 crayons
Maria had 25 crayons left.
50 − 25 = 25
Qi borrowed 25 crayons from Maria.
10. Tina had 3 times as many stickers as Jill while Jack had 4 times as many stickers as Jill.
After Tina gave 10 stickers away and Jack gave 30 stickers to Jill, they each had the same number of stickers.
How many stickers had Jack at first?
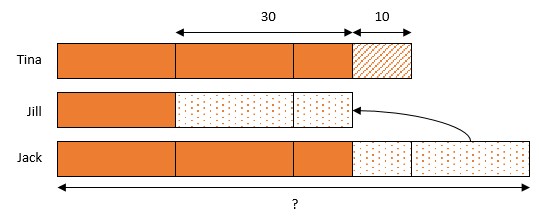 2 units −> 30 + 10 = 40 stickers
2 units −> 30 + 10 = 40 stickers1 unit −> 40 ÷ 2 = 20 stickers
4 units −> 20 × 4 = 80 stickers
Jack had 80 stickers at first.
11. Yana and Zach have an income of $7500 together.
If Zach has an income of $1500, how many times as much income as Zach does Yana have?

$7500 − $1500 = $6000
Yana has an income of $6000. ......(a)
$6000 ÷ $1500 = 4
Yana has 4 times as much income as Zach.
12. A shopkeeper bought some key rings from a supplier.
He threw away 15 broken key rings and sold the rest at 5 for $7.
If he received $427, how many key rings had he bought from the supplier?

$427 ÷ $7 = 61
He sold 61 sets of 5 key rings.
61 × 5 = 305
He sold 605 key rings.
605 + 15 = 620
He had bought 620 key rings from the supplier.
13. A box contains 96 folders.
A bookstore keeper bought 32 boxes of folders and divided the folders into packs of 10.
If he sold each pack for $7 and the remaining folders for $1 each, find the sum of money that the bookstore keeper got.
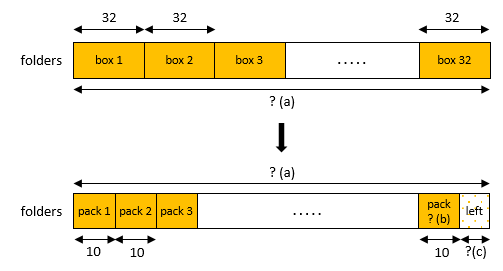
32 × 96 = 3072
He bought 3072 folders altogether.
3072 ÷ 10 = 307 R 2
There were 307 packs of 10 folders each.
There was a remainder of 2 folders.
307 × $7 = $2149
He sold the 307 packs for $2149 altogether.
2 × $1 = $2
He sold the remaining 2 folders for $2 altogether.
$2149 + $2 = $2151
He got $2151 altogether.
14. Mei had 14 rubber bands before she found a packet containing some rubber bands..
She and her 5 friends then shared the rubber bands equally among themselves.
If Mei has 19 rubber bands now, how many rubber bands did the packet have?
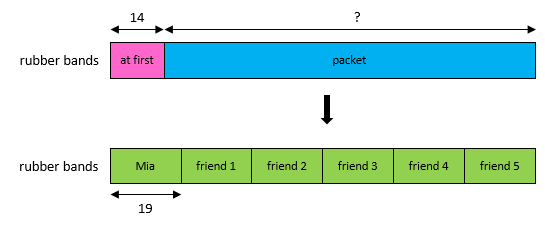
Total number of friends including Mei is 6.
19 × 6 = 114
Total number of rubber bands is 114.
114 − 14 = 100
The packet had 100 rubber bands.
15. Jeremy had 3 times as much money as Ryan.
After Jeremy bought a camera for $179 and Ryan bought a jersey for $38, Ryan had 2 times as much money as Jeremy.
How much money had Jeremy at first?
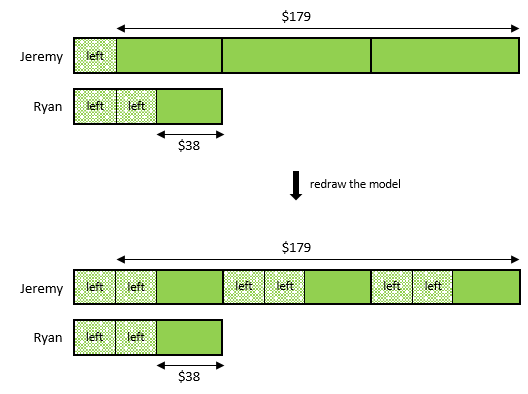
5 units −> $179 − (3 × $38) = $65
1 unit −> $65 ÷ 5 = $13
$179 + $13 = $192
Jeremy had $192 at first.
16. 130 children and 110 adults took a Ferris wheel ride.
If a child ticket cost $9 and an adult ticket cost $16, how much money was collected by the Ferris wheel operator?
130 × $9 = $1170
Sum of money collected from child tickets is $1170.
110 × $16 = $1760
Sum of money collected from adult tickets is $1760.
$1170 + $1760 = $2930
Total sum of money collected by the Ferris wheel operator is $2930.
Sum of money collected from child tickets is $1170.
110 × $16 = $1760
Sum of money collected from adult tickets is $1760.
$1170 + $1760 = $2930
Total sum of money collected by the Ferris wheel operator is $2930.
17. A mom is 3 times as old as her son.
10 years later, the mom will be 2 times as old as the son.
How old is the son now?
METHOD 1: Guess and Check

The son is 10 years old now.
METHOD 2: Model
Watch the video above to understand the steps.
1 unit −> 10 years
The son is 10 years old now.

The son is 10 years old now.
METHOD 2: Model
Watch the video above to understand the steps.
1 unit −> 10 years
The son is 10 years old now.
18. Joe paid $10 000 upfront for a truck priced at $96 400.
He will pay the remaining sum of money by monthly instalments of $600 each.
How many years will it take Joe to pay off all the instalments?
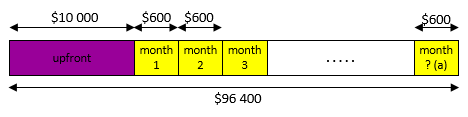
$96 400 − $10 000 = $86 400
The reamining sum of money to be paid is $86 400.
$86 400 ÷ $600 = 144
It will take him 144 months to pay off all the instalments. ......(a)
144 ÷ 12 = 12
It will take him 12 years to pay off all the instalments.
19. A floor measuring 700 cm by 500 cm is being tiled using 100-cm2 tiles.
If each 100-cm2 tile costs $2, how much will it cost to tile the floor?
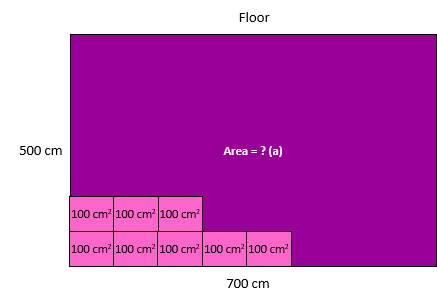
700 × 500 = 350 000
Total area to be tiled is 350 000 cm2. ......(a)

350 000 ÷ 100 = 3500
Number of 100-cm2 tiles needed is 3500. ......(b)
3500 × $2 = $7000
Cost of tiling the floor is $7000.
20. Following are the rates that a cab charges.

How much do you pay for a 4-km ride?
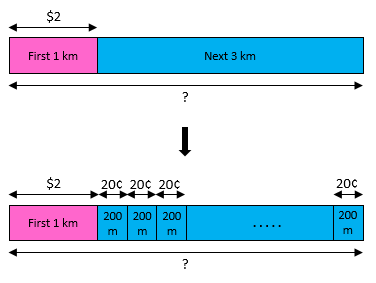
Fare for the first 1 km = $2
4 km − 1 km = 3 km or 3000 m
Remaining distance is 3 km or 3000 m.
3000 m ÷ 200 m = 15
There are 15 sets of 200 m in 3000 m.
15 × 20 ¢ = 300 ¢ or $3
Fare for the reamining distance is $3.
$2 + $3 = $5
You pay $5 for a 4-km ride.
21. Riley types at a speed of 25 words a minute.
If she took 18 minutes to type an essay, how many words did the essay have?
1 min −> 25 words
2 min −> 2 × 25 = 50 words
18 min −> 18 × 25 = 450 words
The essay had 450 words.
2 min −> 2 × 25 = 50 words
18 min −> 18 × 25 = 450 words
The essay had 450 words.
22. The table below shows the number of travelers that arrived at an airport last week.

There were twice as many travelers on Saturday as on Sunday and twice as many on Friday as on Saturday.
How many travelers arrived on Friday?
998 + 498 + 749 + 1500 = 3745
There were 3745 travelers from altogether Monday to Thursday.
16240 − 3745 = 12495
There were 12495 travelers altogether from Friday to Sunday.
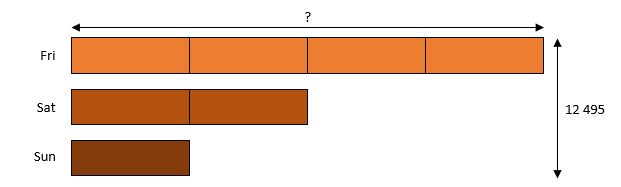
7 units −> 12495
1 unit −> 12495 ÷ 7 = 1785
4 units −> 4 × 1785 = 7140
There were 7140 travelers on Friday.
There were 3745 travelers from altogether Monday to Thursday.
16240 − 3745 = 12495
There were 12495 travelers altogether from Friday to Sunday.
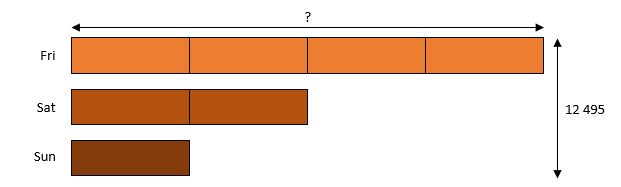
7 units −> 12495
1 unit −> 12495 ÷ 7 = 1785
4 units −> 4 × 1785 = 7140
There were 7140 travelers on Friday.
23. The total mass of a tin containing 16 boxes of cookies is 3260 g.
The mass of the tin is 220 g.
If each empty box has a mass of 10 g, what is the mass of cookies inside each box?
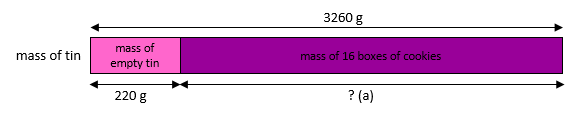
3260 − 220 = 3040
The mass of the 16 boxes of cookies is 3040 g. ......(a)
3040 ÷ 16 = 190
The mass of each box of cookies is 190 g.
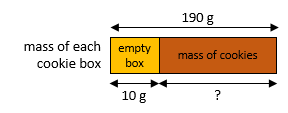
190 − 10 = 180
The mass of cookies inside each box is 180 g.