Finding the Area of a Triangle
Formula for Area of a Triangle
The area of a triangle =
× base × height
| 1 |
| 2 |
1. Deriving the formula to calculate area of a triangle.
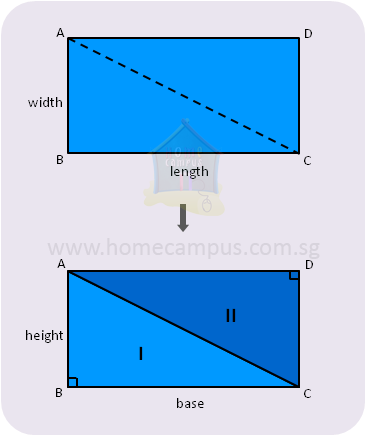
In the figure below,
Area of the rectangle ABCD = length × width
Cut the rectangle into 2.
Now, each half is a right-angled triangle.
And, each right-angled triangle, therefore, has half the area of the rectangle.
Area of right-angled triangle
=
area of rectangle
=
× (length × width)
=
× (base × height)
Area of the rectangle ABCD = length × width
Cut the rectangle into 2.
Now, each half is a right-angled triangle.
And, each right-angled triangle, therefore, has half the area of the rectangle.

Area of right-angled triangle
=
| 1 |
| 2 |
=
| 1 |
| 2 |
=
| 1 |
| 2 |
Study the following examples.
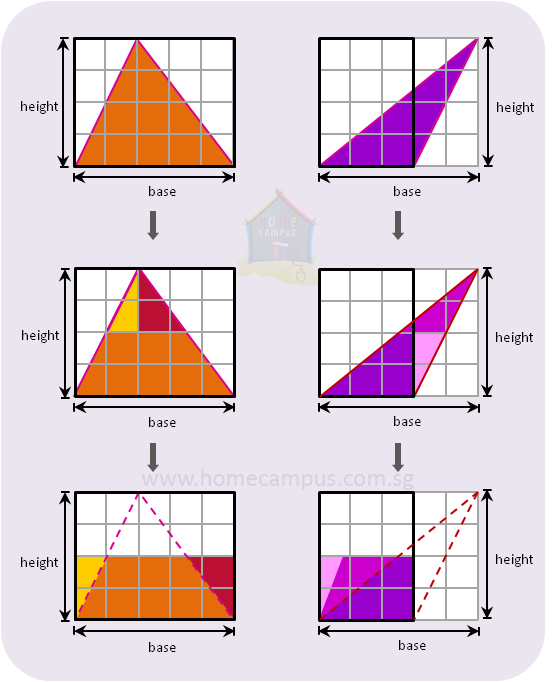
In both examples above, the area of the triangle is half the area of the rectangle made by the base and height.
So,
Area of a triangle
=
of area of rectangle made by the base and height
=
× (base × height)

In both examples above, the area of the triangle is half the area of the rectangle made by the base and height.
So,
Area of a triangle
=
| 1 |
| 2 |
=
| 1 |
| 2 |
2. Find the area of a triangle that has a base of 3 cm and a height of 6 cm.
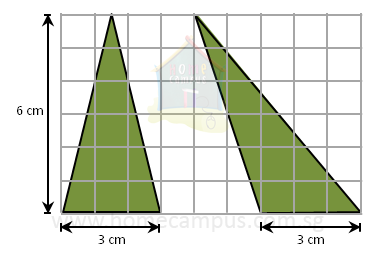
Both triangles above have a base of 3 cm and a height of 6 cm.
Therefore, they both have the same area which is calculated as below:
Area of triangle
=
| 1 |
| 2 |
=
| 1 |
| 2 |
= 9 cm2
3. Find the area of the shaded pentagon below.

Area of triangle ABC
=
| 1 |
| 2 |
=
| 1 |
| 2 |
= 10 cm2
Area of triangle BED
=
| 1 |
| 2 |
= 0.75 cm2
Area of triangle GFC
=
| 1 |
| 2 |
= 2.25 cm2
Area of pentagon ADEFG
= Area (ABC) − Area (BED) − Area (GFC)
= 10 cm2 − 0.75 cm2 − 2.25 cm2
= 7 cm2